Section 4.4 : Hyperbolas
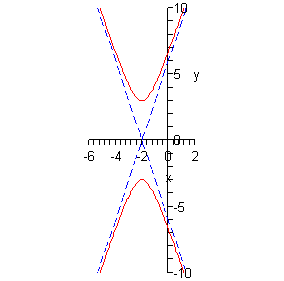
The next graph that we need to look at is the hyperbola. There are two basic forms of a hyperbola. Here are examples of each.
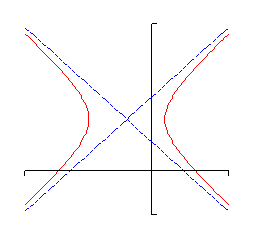
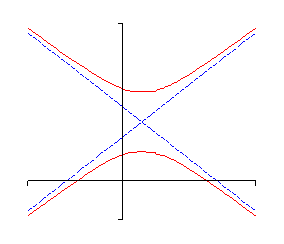
Hyperbolas consist of two vaguely parabola shaped pieces that open either up and down or right and left. Also, just like parabolas each of the pieces has a vertex. Note that they aren’t really parabolas, they just resemble parabolas.
There are also two lines on each graph. These lines are called asymptotes and as the graphs show as we make x large (in both the positive and negative sense) the graph of the hyperbola gets closer and closer to the asymptotes. The asymptotes are not officially part of the graph of the hyperbola. However, they are usually included so that we can make sure and get the sketch correct. The point where the two asymptotes cross is called the center of the hyperbola.
There are two standard forms of the hyperbola, one for each type shown above. Here is a table giving each form as well as the information we can get from each one.
| Form | (x−h)2a2−(y−k)2b2=1 | (y−k)2b2−(x−h)2a2=1 | |
| Center | (h,k) | (h,k) | |
| Opens | Opens left and right | Opens up and down | |
| Vertices | (h+a,k) and (h−a,k) | (h,k+b) and (h,k−b) | |
| Slope of Asymptotes | ±ba | ±ba | |
| Equations of Asymptotes | y=k±ba(x−h) | y=k±ba(x−h) | |
Note that the difference between the two forms is which term has the minus sign. If the y term has the minus sign then the hyperbola will open left and right. If the x term has the minus sign then the hyperbola will open up and down.
We got the equations of the asymptotes by using the point-slope form of the line and the fact that we know that the asymptotes will go through the center of the hyperbola.
Let’s take a look at a couple of these.
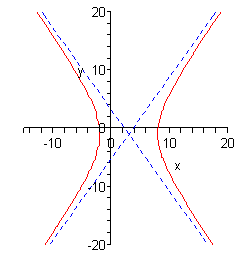
- (x−3)225−(y+1)249=1
- y29−(x+2)2=1
Now, notice that the y term has the minus sign and so we know that we’re in the first column of the table above and that the hyperbola will be opening left and right.
The first thing that we should get is the center since pretty much everything else is built around that. The center in this case is (3,−1) and as always watch the signs! Once we have the center we can get the vertices. These are (8,−1) and (−2,−1).
Next, we should get the slopes of the asymptotes. These are always the square root of the number under the y term divided by the square root of the number under the x term and there will always be a positive and a negative slope. The slopes are then ±75.
Now that we’ve got the center and the slopes of the asymptotes we can get the equations for the asymptotes. They are,
y=−1+75(x−3)andy=−1−75(x−3)We can now start the sketching. We start by sketching the asymptotes and the vertices. Once these are done we know what the basic shape should look like so we sketch it in making sure that as x gets large we move in closer and closer to the asymptotes.
Here is the sketch for this hyperbola.
b y29−(x+2)2=1 Show Solution
In this case the hyperbola will open up and down since the x term has the minus sign. Now, the center of this hyperbola is (−2,0). Remember that since there is a y2 term by itself we had to have k=0. At this point we also know that the vertices are (−2,3) and (−2,−3).
In order to see the slopes of the asymptotes let’s rewrite the equation a little.
y29−(x+2)21=1So, the slopes of the asymptotes are ±31=±3. The equations of the asymptotes are then,
y=0+3(x+2)=3x+6and y=0−3(x+2)=−3x−6Here is the sketch of this hyperbola.