Calculus III - Surface Area

Show Mobile Notice

Mobile Notice

You appear to be on a device with a "narrow" screen width (i.e. you are probably on a mobile phone). Due to the nature of the mathematics on this site it is best viewed in landscape mode. If your device is not in landscape mode many of the equations will run off the side of your device (you should be able to scroll/swipe to see them) and some of the menu items will be cut off due to the narrow screen width.

Section 15.9 : Surface Area

In this section we will look at the lone application (aside from the area and volume interpretations) of multiple integrals in this material. This is not the first time that we’ve looked at surface area We first saw surface area in Calculus II, however, in that setting we were looking at the surface area of a solid of revolution. In other words, we were looking at the surface area of a solid obtained by rotating a function about the $x$ or $y$ axis. In this section we want to look at a much more general setting although you will note that the formula here is very similar to the formula we saw back in Calculus II.

Here we want to find the surface area of the surface given by $z = f\left( {x,y} \right)$ where $\left( {x,y} \right)$ is a point from the region $D$ in the $xy$ -plane. In this case the surface area is given by,

S = \iint D \sqrt{{[f_{x}]}^{2} + {[f_{y}]}^{2} + 1} d A

$S = \iint\limits_{D}{{\sqrt {\,{{\left[ {{f_x}} \right]}^2} + {{\left[ {{f_y}} \right]}^2} + 1} \,dA}}$

Let’s take a look at a couple of examples.

Example 1 Find the surface area of the part of the plane

3 x + 2 y + z = 6

$3x + 2y + z = 6$ that lies in the first octant.

Show Solution

Remember that the first octant is the portion of the xyz-axis system in which all three variables are positive. Let’s first get a sketch of the part of the plane that we are interested in.

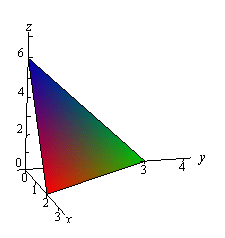
This is a graph with the standard 3D coordinate system. The positive z-axis is straight up, the positive x-axis moves downwards and slightly to the right and positive y-axis moves off nearly horizontal to the right. This is the graph in the 1st octant of the plane given in the problem statement. It is a triangle with vertices (0,0,6), (2,0,0) and (0,3,0).

This is a boxed 3D coordinate system. The z-axis is right vertical edge of the box, the x-axis is the top right edge of the box and the y-axis is the bottom front edge of the box. This is the graph in the 1st octant of the plane given in the problem statement. It is a triangle with vertices (0,0,6), (2,0,0) and (0,3,0).

We’ll also need a sketch of the region $D$ .

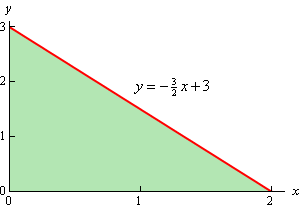
$This is the 2D graph on a xy axis system of a triangle with vertices (0,0), (2,0) and (0,3). The top left edge of the triangle is given by $y=-\frac{3}{2}x+3$. The bottom of the triangle is the x-axis and the left edge is the y-axis. The triangle has been shaded in.$

Remember that to get the region $D$ we can pretend that we are standing directly over the plane and what we see is the region $D$ . We can get the equation for the hypotenuse of the triangle by realizing that this is nothing more than the line where the plane intersects the $xy$ -plane and we also know that $z = 0$ on the $xy$ -plane. Plugging $z = 0$ into the equation of the plane will give us the equation for the hypotenuse.

Notice that in order to use the surface area formula we need to have the function in the form $z = f\left( {x,y} \right)$ and so solving for $z$ and taking the partial derivatives gives,

z = 6 - 3 x - 2 y f_{x} = - 3 f_{y} = - 2

$z = 6 - 3x - 2y\hspace{0.5in}{f_x} = - 3\hspace{0.25in}{f_y} = - 2$

The limits defining $D$ are,

0 \leq x \leq 20 \leq y \leq - \frac{3}{2} x + 3

$0 \le x \le 2\hspace{0.5in}0 \le y \le - \frac{3}{2}x + 3$

The surface area is then,

\begin{matrix} S & = \iint D \sqrt{{[- 3]}^{2} + {[- 2]}^{2} + 1} d A = \int_{0}^{2} \int_{0}^{- \frac{3}{2} x + 3} \sqrt{14} d y d x = \sqrt{14} \int_{0}^{2} - \frac{3}{2} x + 3 d x = \sqrt{14} {(- \frac{3}{4} x^{2} + 3 x) ∣ ∣ ∣}_{0}^{2} = 3 \sqrt{14} \end{matrix}

$\begin{align*}S & = \iint\limits_{D}{{\sqrt {\,{{\left[ { - 3} \right]}^2} + {{\left[ { - 2} \right]}^2} + 1} \,dA}}\\ & = \int_{{\,0}}^{{\,2}}{{\int_{{\,0}}^{{\, - \frac{3}{2}x + 3}}{{\sqrt {14} \,dy}}\,dx}}\\ & = \sqrt {14} \int_{{\,0}}^{{\,2}}{{ - \frac{3}{2}x + 3\,dx}}\\ & = \sqrt {14} \left. {\left( { - \frac{3}{4}{x^2} + 3x} \right)} \right|_0^2\\ & = 3\sqrt {14} \end{align*}$

Example 2 Determine the surface area of the part of

z = x y

$z = xy$ that lies in the cylinder given by

x^{2} + y^{2} = 1

${x^2} + {y^2} = 1$ .

Show Solution

In this case we are looking for the surface area of the part of $z = xy$ where $\left( {x,y} \right)$ comes from the disk of radius 1 centered at the origin since that is the region that will lie inside the given cylinder.

Here are the partial derivatives,

f_{x} = y f_{y} = x

${f_x} = y\hspace{0.5in}{f_y} = x$

The integral for the surface area is,

S = \iint D \sqrt{x^{2} + y^{2} + 1} d A

$S = \iint\limits_{D}{{\sqrt {\,{x^2} + {y^2} + 1} \,dA}}$

Given that $D$ is a disk it makes sense to do this integral in polar coordinates.

\begin{matrix} S & = \iint D \sqrt{x^{2} + y^{2} + 1} d A = \int_{0}^{2 π} \int_{0}^{1} r \sqrt{1 + r^{2}} d r d θ = \int_{0}^{2 π} {\frac{1}{2} (\frac{2}{3}) {(1 + r^{2})}^{\frac{3}{2}} ∣ ∣ ∣}_{0}^{1} d θ = \int_{0}^{2 π} \frac{1}{3} (2^{\frac{3}{2}} - 1) d θ = \frac{2 π}{3} (2^{\frac{3}{2}} - 1) \end{matrix}

$\begin{align*}S & = \iint\limits_{D}{{\sqrt {\,{x^2} + {y^2} + 1} \,dA}}\\ & = \int_{{\,0}}^{{\,2\pi }}{{\int_{{\,0}}^{{\,1}}{{r\sqrt {1 + {r^2}} \,dr}}\,d\theta }}\\ & = \int_{{\,0}}^{{\,2\pi }}{{\left. {\frac{1}{2}\left( {\frac{2}{3}} \right){{\left( {1 + {r^2}} \right)}^{\frac{3}{2}}}} \right|_0^1\,d\theta }}\\ & = \int_{{\,0}}^{{\,2\pi }}{{\frac{1}{3}\left( {{2^{\frac{3}{2}}} - 1} \right)\,d\theta }}\\ & = \frac{{2\pi }}{3}\left( {{2^{\frac{3}{2}}} - 1} \right)\end{align*}$