Section 6.6 : Work
This is the final application of integral that we’ll be looking at in this course. In this section we will be looking at the amount of work that is done by a force in moving an object.
In a first course in Physics you typically look at the work that a constant force, F, does when moving an object over a distance of d. In these cases the work is,
W=FdHowever, most forces are not constant and will depend upon where exactly the force is acting. So, let’s suppose that the force at any x is given by F(x). Then the work done by the force in moving an object from x=a to x=b is given by,
W=∫baF(x)dxTo see a justification of this formula see the Proof of Various Integral Properties section of the Extras chapter.
Notice that if the force is constant we get the correct formula for a constant force.
W=∫baFdx=Fx|ba=F(b−a)where b−a is simply the distance moved, or d.
So, let’s take a look at a couple of examples of non-constant forces.
This example will require Hooke’s Law to determine the force. Hooke’s Law tells us that the force required to stretch a spring a distance of x meters from its natural length is,
F(x)=kxwhere k>0 is called the spring constant. It is important to remember that the x in this formula is the distance the spring is stretched from its natural length and not the actual length of the spring.
So, the first thing that we need to do is determine the spring constant for this spring. We can do that using the initial information. A force of 40 N is required to stretch the spring
30cm−20cm=10cm=0.1mfrom its natural length. Using Hooke’s Law we have,
40=0.10k⇒k=400So, according to Hooke’s Law the force required to hold this spring x meters from its natural length is,
F(x)=400xWe want to know the work required to stretch the spring from 35cm to 38cm. First, we need to convert these into distances from the natural length in meters. Doing that gives us x’s of 0.15m and 0.18m.
The work is then,
W=∫0.180.15400xdx=200x2|0.180.15=1.98J- Determine the amount of work required to lift the bucket to the midpoint of the shaft.
- Determine the amount of work required to lift the bucket from the midpoint of the shaft to the top of the shaft.
- Determine the amount of work required to lift the bucket all the way up the shaft.
Before answering either part we first need to determine the force. In this case the force will be the weight of the bucket and cable at any point in the shaft.
To determine a formula for this we will first need to set a convention for x. For this problem we will set x to be the amount of cable that has been pulled up. So at the bottom of the shaft x=0, at the midpoint of the shaft x=250 and at the top of the shaft x=500. Also, at any point in the shaft there is 500−x feet of cable still in the shaft.
The force then for any x is then nothing more than the weight of the cable and bucket at that point. This is,
F(x)=weight of cable+ weight of bucket/coal=2(500−x)+800=1800−2xWe can now answer the questions.
a Determine the amount of work required to lift the bucket to the midpoint of the shaft. Show Solution
In this case we want to know the work required to move the cable and bucket/coal from x=0 to x=250. The work required is,
W=∫2500F(x)dx=∫25001800−2xdx=(1800x−x2)|2500=387500ft - lbb Determine the amount of work required to lift the bucket from the midpoint of the shaft to the top of the shaft. Show Solution
In this case we want to move the cable and bucket/coal from x=250 to x=500. The work required is,
W=∫500250F(x)dx=∫5002501800−2xdx=(1800x−x2)|500250=262500ft - lbc Determine the amount of work required to lift the bucket all the way up the shaft. Show Solution
In this case the work is,
W=∫5000F(x)dx=∫50001800−2xdx=(1800x−x2)|5000=650000ft - lbNote that we could have instead just added the results from the first two parts and we would have gotten the same answer to the third part.
First, we need to determine the weight per foot of the cable. This is easy enough to get,
80 lbs20 ft=4 lb/ftNext, let x be the distance from the ceiling to any point on the cable. Using this convention we can see that the portion of the cable in the range 10<x≤20 will actually be lifted. The portion of the cable in the range 0≤x≤10 will not be lifted at all since once the bottom of the cable has been lifted up to the ceiling the cable will be doubled up and each portion will have a length of 10 ft. So, the upper 10 foot portion of the cable will never be lifted while the lower 10 ft portion will be lifted.
Now, the very bottom of the cable, x=20, will be lifted 10 feet to get to the midpoint and then a further 10 feet to get to the ceiling. A point 2 feet from the bottom of the cable, x=18 will lift 8 feet to get to the midpoint and then a further 8 feet until it reaches its final position (if it is 2 feet from the bottom then its final position will be 2 feet from the ceiling). Continuing on in this fashion we can see that for any point on the lower half of the cable, i.e. 10≤x≤20 it will be lifted a total of 2(x−10).
As with the previous example the force required to lift any point of the cable in this range is simply the distance that point will be lifted times the weight/foot of the cable. So, the force is then,
F(x)=(distance lifted)(weight per foot of cable)=2(x−10)(4)=8(x−10)The work required is now,
W=∫20108(x−10)dx=(4x2−80x)|2010=400ft - lbProvided we can find the force, F(x), for a given problem then using the above method for determining the work is (generally) pretty simple. However, there are some problems where this approach won’t easily work. Let’s take a look at one of those kinds of problems.
Okay, in this case we cannot just determine a force function, F(x) that will work for us. So, we are going to need to approach this from a different standpoint.
Let’s first set x=0 to be the lower end of the tank/cone and x=15 to be the top of the tank/cone. With this definition of our x’s we can now see that the water in the tank will correspond to the interval [0,12].
So, let’s start off by dividing [0,12] into n subintervals each of width Δx and let’s also let x∗i be any point from the ith subinterval where i=1,2,…n. Now, for each subinterval we will approximate the water in the tank corresponding to that interval as a cylinder of radius ri and height Δx.
Here is a quick sketch of the tank. Note that the sketch really isn’t to scale and we are looking at the tank from directly in front so we can see all the various quantities that we need to work with.
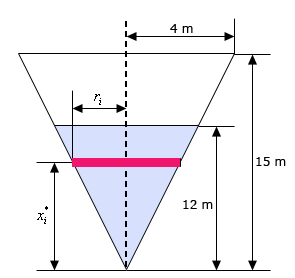
The red strip in the sketch represents the “cylinder” of water in the ith subinterval. A quick application of similar triangles will allow us to relate ri to x∗i (which we’ll need in a bit) as follows.
rix∗i=415⇒ri=415x∗iOkay, the mass, mi, of the volume of water, Vi, for the ith subinterval is simply,
mi=density ×ViWe know the density of the water (it was given in the problem statement) and because we are approximating the water in the ith subinterval as a cylinder we can easily approximate the volume as,
Vi≈π(radius)2(height)We can now approximate the mass of water in the ith subinterval,
mi≈(1000)[πr2iΔx]=1000π(415x∗i)2Δx=6409π(x∗i)2ΔxTo raise this volume of water we need to overcome the force of gravity that is acting on the volume and that is, F=mig, where g=9.8m/s2 is the gravitational acceleration. The force to raise the volume of water in the ith subinterval is then approximately,
Fi=mig≈(9.8)6409π(x∗i)2ΔxNext, in order to reach to the top of the tank the water in the ith subinterval will need to travel approximately 15−x∗i to reach the top of the tank. Because the volume of the water in the ith subinterval is constant the force needed to raise the water through any distance is also a constant force.
Therefore, the work to move the volume of water in the ith subinterval to the top of the tank, i.e. raise it a distance of 15−x∗i, is then approximately,
Wi≈Fi(15−x∗i)=(9.8)6409π(x∗i)2(15−x∗i)ΔxThe total amount of work required to raise all the water to the top of the tank is then approximately the sum of each of the Wi for i=1,2,…n. Or,
W≈n∑i=1(9.8)6409π(x∗i)2(15−x∗i)ΔxTo get the actual amount of work we simply need to take n→∞. I.e. compute the following limit,
W=limThis limit of a summation should look somewhat familiar to you. It’s probably been some time, but recalling the definition of the definite integral we can see that this is nothing more than the following definite integral,
\begin{align*}W &= \int_{0}^{{12}}{{\left( {9.8} \right)\frac{{640}}{9}\pi {x^2}\left( {15 - x} \right)\,dx}} = \left( {9.8} \right)\frac{{640}}{9}\pi \int_{0}^{{12}}{{15{x^2} - {x^3}\,dx}}\\ & = \left. {\left( {9.8} \right)\frac{{640}}{9}\pi \left( {5{x^3} - \frac{1}{4}{x^4}} \right)} \right|_0^{12} = 7,566,362.543\,{\mbox{J}}\end{align*}As we’ve seen in the previous example we sometimes need to compute “incremental” work and then use that to determine the actual integral we need to compute. This idea does arise on occasion and we shouldn’t forget it!