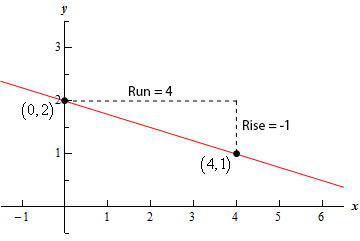Section 3.2 : Lines
6. Determine the slope of the line and sketch the graph of the following line.
4y+x=8Show All Steps Hide All Steps
Start SolutionThe first thing that we should do here is write the equation of the line in slope-intercept form. This will help with both finding the slope and with sketching the graph.
Here is the slope-intercept form of the line.
4y=−x+8⇒y=−14x+2 Show Step 2From the equation of the line in slope-intercept form that we found in the previous step we see that the slope is : −14 .
Show Step 3From the equation of line in slope-intercept form that we found in Step 1 we see that the y-intercept from of the line is (0,2) . Also, from the slope we found in Step 2 we know that the “rise” is -1 and the “run” is 4 and so a second point on the graph of the line is,
x2=0+4=4y2=2+(−1)=1⇒(4,1)Using these two points we can sketch the graph of the line.