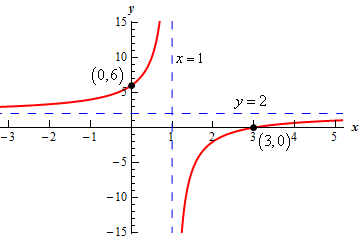Section 4.8 : Rational Functions
2. Sketch the graph of the following function. Clearly identify all intercepts and asymptotes.
f(x)=6−2x1−xShow All Steps Hide All Steps
Start SolutionLet’s first find the intercepts for this function.
The y-intercept is the point (0,f(0))=(0,6).
For the x-intercepts we set the numerator equal to zero and solve. Doing that for this problem gives,
6−2x=0→x=3So, the only x-intercept for this problem is (3,0).
Show Step 2We can find any vertical asymptotes be setting the denominator equal to zero and solving. Doing that for this function gives,
1−x=0→x=1So, we’ll have a vertical asymptote at x=1.
Show Step 3For this equation the largest exponent of x in both the numerator and denominator is 1. Therefore, the horizontal asymptote for this problem is then the coefficient of the x in the numerator divided by the coefficient of the x in the denominator. Or,
y=−2−1=2 Show Step 4From Step 2 we saw we only have one vertical asymptote and so we only have two regions to our graph : x<1 and x>1.
We’ll need a point in each region to determine if it will be above or below the horizontal asymptote. Here are a couple of function evaluations for the points.
f(0)=6→(0,6)f(3)=0→(3,0)Note that both of these are the intercepts we found in the first step. In this case they both just happened to be on either side of the vertical asymptote and so we could use these two points here.
Show Step 5Here is a sketch of the function with the points found above. The vertical and horizontal asymptotes are indicated with blue dashed lines.