Section 4.5 : The Shape of a Graph, Part I
5. For f(x)=2x3−9x2−60x answer each of the following questions.
- Identify the critical points of the function.
- Determine the intervals on which the function increases and decreases.
- Classify the critical points as relative maximums, relative minimums or neither.
Show All Solutions Hide All Solutions
a Identify the critical points of the function. Show SolutionWe need the 1st derivative to get the critical points so here it is.
f′(x)=6x2−18x−60=6(x2−3x−10)=6(x−5)(x+2)Now, recall that critical points are where the derivative doesn’t exist or is zero. Clearly this derivative exists everywhere (it’s a polynomial….) and because we factored the derivative we can easily identify where the derivative is zero. The critical points of the function are,
\require{bbox} \bbox[2pt,border:1px solid black]{{x = - 2,\,\,\,\,\,\,\,\,x = 5}}b Determine the intervals on which the function increases and decreases. Show Solution
To determine the increase/decrease information for the function all we need is a quick number line for the derivative. Here is the number line.
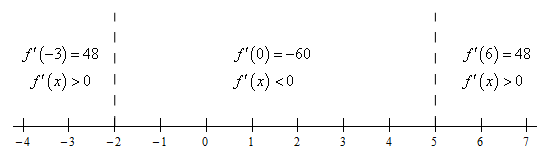
From this we get the following increasing/decreasing information for the function.
\require{bbox} \bbox[2pt,border:1px solid black]{{{\mbox{Increasing : }}\left( { - \infty , - 2} \right)\,\,\,\,\& \,\,\,\left( {5,\infty } \right)\hspace{0.25in}\hspace{0.25in}{\mbox{Decreasing : }}\,\,\left( { - 2,5} \right)}}c Classify the critical points as relative maximums, relative minimums or neither. Show Solution
With the increasing/decreasing information from the previous step we can easily classify the critical points using the 1st derivative test. Here is classification of the functions critical points.
\require{bbox} \bbox[2pt,border:1px solid black]{\begin{align*}x & = - 2 : {\mbox{ Relative Maximum}}\\ x & = 5:{\mbox{ Relative Minimum}}\end{align*}}