Section 15.9 : Surface Area
3. Determine the surface area of the portion of z=3+2y+14x4 that is above the region in the xy-plane bounded by y=x5, x=1 and the x-axis.
Show All Steps Hide All Steps
Start SolutionOkay, let’s start off with a quick sketch of the surface so we can get a feel for what we’re dealing with.
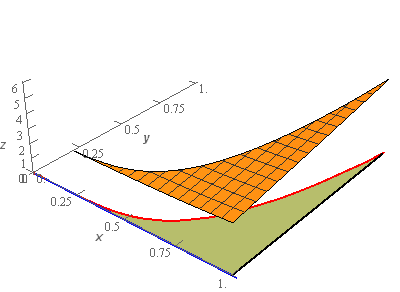
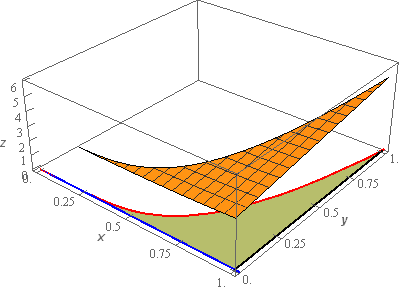
We’ve given the sketches with a set of “traditional” axes as well as a set of “box” axes to help visualize the surface.
The surface we are after is the orange portion that is above the xy-plane and the greenish region in the xy-plane is the region over which we are graphing the surface, i.e. it is the region D we’ll use in the integral.
Show Step 2The integral for the surface area is,
S=∬ Show Step 3Now, as mentioned in Step 1 the region D is shown in the sketches of the surface. Here is a 2D sketch of D for the sake of completeness.
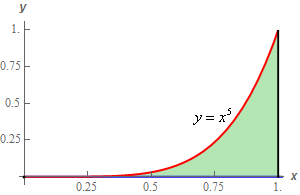
The limits for this region are,
\begin{array}{c}0 \le x \le 1\\ 0 \le y \le {x^5}\end{array}Note as well that the integrand pretty much requires us to do the integration in this order.
Show Step 4With the limits from Step 3 the integral becomes,
S = \iint\limits_{D}{{\sqrt {{x^6} + 5} \,dA}} = \int_{0}^{1}{{\int_{0}^{{{x^5}}}{{\sqrt {{x^6} + 5} \,dy}}\,dx}} Show Step 5Okay, all we need to do then is evaluate the integral.
\begin{align*}S & = \int_{0}^{1}{{\int_{0}^{{{x^5}}}{{\sqrt {{x^6} + 5} \,dy}}\,dx}}\\ & = \int_{0}^{1}{{\left. {\left( {y\sqrt {{x^6} + 5} } \right)} \right|_0^{{x^5}}\,dx}}\\ & = \int_{0}^{1}{{{x^5}\sqrt {{x^6} + 5} \,dx}}\\ & = \left. {\frac{1}{9}{{\left( {{x^6} + 5} \right)}^{\frac{3}{2}}}} \right|_0^1 = \require{bbox} \bbox[2pt,border:1px solid black]{{\frac{1}{9}\left( {{6^{\frac{3}{2}}} - {5^{\frac{3}{2}}}} \right) = 0.3907}}\end{align*}