Section 15.9 : Surface Area
4. Determine the surface area of the portion of y=2x2+2z2−7that is inside the cylinder x2+z2=4.
Show All Steps Hide All Steps
Start SolutionOkay, let’s start off with a quick sketch of the surface so we can get a feel for what we’re dealing with.
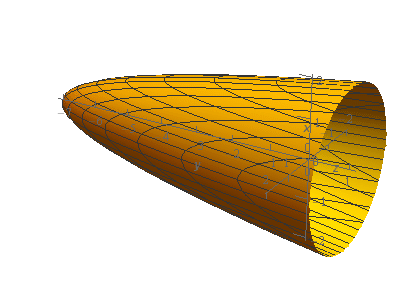
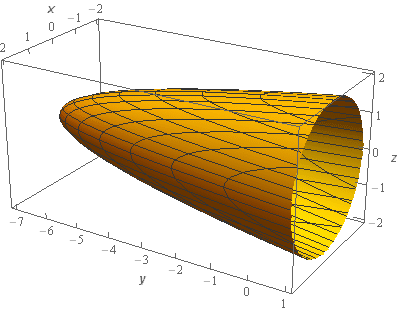
We’ve given the sketches with a set of “traditional” axes as well as a set of “box” axes to help visualize the surface.
So, we have an elliptic paraboloid centered on the y-axis. This also means that the region D for our integral will be in the xz-plane and we’ll be needing polar coordinates for the integral.
Show Step 2As noted above the region D is going to be in the xz-plane and the surface is given in the form y=f(x,z). The formula for surface area we gave in the notes is only for a region D that is in the xy-plane with the surface given by z=f(x,y) .
However, it shouldn’t be too difficult to see that all we need to do is modify the formula in the following manner to get one for this setup.
S=∬D√[fx]2+[fz]2+1dAy=f(x,z)Dis in the xz - planeSo, the integral for the surface area is,
S=∬D√[4x]2+[4z]2+1dA=∬D√16x2+16z2+1dA Show Step 3Now, as we noted in Step 1 the region D is in the xz-plane and because we are after the portion of the elliptical paraboloid that is inside the cylinder given by x2+z2=4 we can see that the region D must therefore be the disk x2+z2≤4.
Also as noted in Step 1 we’ll be needing polar coordinates for this integral so here are the limits for the integral in terms of polar coordinates.
0≤θ≤2π0≤r≤2 Show Step 4Now, let’s convert the integral into polar coordinates. However, they won’t be the “standard” polar coordinates. Because D is in the xz-plane we’ll need to use the following “modified” polar coordinates.
x=rcosθz=rsinθx2+z2=r2Converting the integral to polar coordinates then gives,
S=∬D√16x2+16z2+1dA=∫2π0∫20r√16r2+1drdθDon’t forget to pick up the extra r from converting the dA into polar coordinates. It is the same dA as we use for the “standard” polar coordinates.
Show Step 5Okay, all we need to do then is evaluate the integral.
S=∫2π0∫20r√16r2+1drdθ=∫2π0148(16r2+1)32|20dθ=∫2π0148(6532−1)dθ=148(6532−1)θ|2π0=π24(6532−1)=68.4667