Section 3.5 : Graphing Functions
4. Construct a table of at least 4 ordered pairs of points on the graph of the following function and use the ordered pairs from the table to sketch the graph of the function.
f(x)={10−2xif x<2x2+2if x≥2Show All Steps Hide All Steps
We have a piecewise function here so we need to make sure and pick points from each range of x when building our table of points. Also, we’ll want to plug the “cutoff point”, x=2, into the top equation as well so we know where it will be at the end of the range of x’s for which it is valid.
Here is the table of points we’ll use for this problem.
| x | 10−2x | (x,y) |
|---|---|---|
| -1 | 12 | (−1,12) |
| 1 | 8 | (1,8) |
| 2 | 6 | (2,6) |
| x | x2+2 | (x,y) |
|---|---|---|
| 2 | 6 | (2,6) |
| 3 | 11 | (3,11) |
| 5 | 27 | (5,27) |
Here is a sketch of the function.
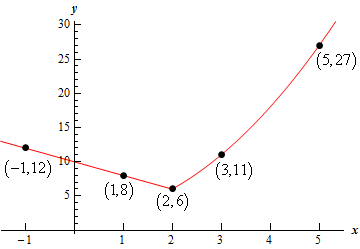
Note that in this case the two pieces “met” at the point (2,6). Sometimes this will happen with these problems and sometimes it won’t. This is the reason we always evaluate both equations at the “cutoff point”. Without doing that we wouldn’t know if the pieces meet or not.