Section 3.2 : Interpretation of the Derivative
1. Use the graph of the function, f(x), estimate the value of f′(a) for
- a=−2
- a=3

Show All Solutions Hide All Solutions
a a=−2 Show All Steps Hide All StepsStart Solution
Given that one of the interpretations of the derivative is that it is the slope of the tangent line to the function at a particular point let’s first sketch in a tangent line at the point on the graph.
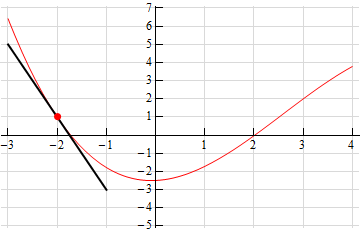
The function is clearly decreasing here and so we know that the derivative at this point will be negative. Now, from this sketch of the tangent line it looks like if we run over 1 we go down 4 and so we can estimate that,
f′(−2)=−4b a=3 Show All Steps Hide All Steps
Start Solution
Given that one of the interpretations of the derivative is that it is the slope of the tangent line to the function at a particular point. Let’s first sketch in a tangent line at the point.
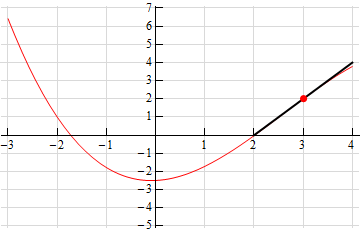
The function is clearly increasing here and so we know that the derivative at this point will be positive. Now, from this sketch of the tangent line it looks like if we run over 1 we go up 2 and so we can estimate that,
f′(3)=2