Calculus I - Infinite Limits

Hide Mobile Notice

Mobile Notice

You appear to be on a device with a "narrow" screen width (i.e. you are probably on a mobile phone). Due to the nature of the mathematics on this site it is best viewed in landscape mode. If your device is not in landscape mode many of the equations will run off the side of your device (you should be able to scroll/swipe to see them) and some of the menu items will be cut off due to the narrow screen width.

Section 2.6 : Infinite Limits

Back to Problem List

2. For $\displaystyle h\left( t \right) = \frac{{2t}}{{6 + t}}$ evaluate,

$\mathop {\lim }\limits_{t \to \, - {6^{\, - }}} h\left( t \right)$
$\mathop {\lim }\limits_{t \to \, - {6^{\, + }}} h\left( t \right)$
$\mathop {\lim }\limits_{t \to \, - 6} h\left( t \right)$

Show All Solutions Hide All Solutions

$\mathop {\lim }\limits_{t \to \, - {6^{\, - }}} h\left( t \right)$ Show Solution

Let’s start off by acknowledging that for $t \to - {6^ - }$ we know $t < - 6$ .

For the numerator we can see that, in the limit, we will get -12.

The denominator takes a little more work. Clearly, in the limit, we have,

$6 + t \to 0$

but we can actually go a little farther. Because we know that $t < - 6$ we also know that,

$6 + t < 0$

More compactly, we can say that in the limit we will have,

$6 + t \to {0^ - }$

So, in the limit, the numerator is approaching a negative number and the denominator is an increasingly small negative number. The quotient must then be an increasing large positive number or,

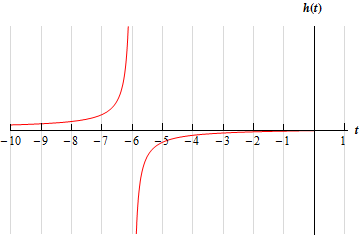
$\require{bbox} \bbox[2pt,border:1px solid black]{{\mathop {\lim }\limits_{t \to \, - {6^{\, - }}} \frac{{2t}}{{6 + t}} = \infty }}$

Note that this also means that there is a vertical asymptote at $t = - 6$ .

$\mathop {\lim }\limits_{t \to \, - {6^{\, + }}} h\left( t \right)$ Show Solution

Let’s start off by acknowledging that for $t \to - {6^ + }$ we know $t > - 6$ .

For the numerator we can see that, in the limit, we will get -12.

The denominator will also work similarly to the first part. In the limit, we have,

$6 + t \to 0$

but we can actually go a little farther. Because we know that $t > - 6$ we also know that,

$6 + t > 0$

More compactly, we can say that in the limit we will have,

$6 + t \to {0^ + }$

So, in the limit, the numerator is approaching a negative number and the denominator is an increasingly small positive number. The quotient must then be an increasing large negative number or,

$\require{bbox} \bbox[2pt,border:1px solid black]{{\mathop {\lim }\limits_{t \to \, - {6^{\, + }}} \frac{{2t}}{{6 + t}} = - \infty }}$

Note that this also means that there is a vertical asymptote at $t = - 6$ , which we already knew from the first part.

$\mathop {\lim }\limits_{t \to \, - 6} h\left( t \right)$ Show Solution

In this case we can see from the first two parts that,

$\mathop {\lim }\limits_{t \to \, - {6^{\, - }}} h\left( t \right) \ne \mathop {\lim }\limits_{t \to \, - {6^{\, + }}} h\left( t \right)$

and so, from our basic limit properties we can see that $\mathop {\lim }\limits_{t \to \, - 6} h\left( t \right)$ does not exist.

For the sake of completeness and to verify the answers for this problem here is a quick sketch of the function.