Section 4.6 : The Shape of a Graph, Part II
3. For f(x)=12+6x2−x3 answer each of the following questions.
- Determine a list of possible inflection points for the function.
- Determine the intervals on which the function is concave up and concave down.
- Determine the inflection points of the function.
Show All Solutions Hide All Solutions
a Determine a list of possible inflection points for the function. Show SolutionTo get the list of possible inflection points for the function we’ll need the 2nd derivative of the function so here that is.
f′(x)=12x−3x2f″Now, recall that possible inflection points are where the 2nd derivative either doesn’t exist or is zero. Clearly the 2nd derivative exists everywhere (it’s a polynomial….) and, in this case, it should be fairly clear where the 2nd derivative is zero. The only possible inflection critical point of the function in this case is,
\underline {x = 2}b Determine the intervals on which the function is concave up and concave down. Show Solution
There isn’t much to this part. All we really need here is a number line for the 2nd derivative. Here that is,
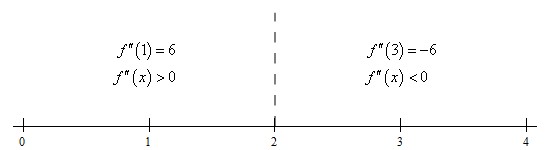
From this we get the following concave up/concave down information for the function.
\require{bbox} \bbox[2pt,border:1px solid black]{{{\mbox{Concave Up : }}\left( { - \infty ,2} \right)\hspace{0.5in}\hspace{0.5in}{\mbox{Concave Down : }}\left( {2,\infty } \right)}}c Determine the inflection points of the function. Show Solution
For this part all we need to do is interpret the results from the previous step. Recall that inflection points are points where the concavity changes (as opposed to simply the points where the 2nd derivative is zero or doesn’t exist). Therefore, the single inflection point for this function is,
\require{bbox} \bbox[2pt,border:1px solid black]{{x = 2}}