Mobile Notice
You appear to be on a device with a "narrow" screen width (i.e. you are probably on a mobile phone). Due to the nature of the mathematics on this site it is best viewed in landscape mode. If your device is not in landscape mode many of the equations will run off the side of your device (you should be able to scroll/swipe to see them) and some of the menu items will be cut off due to the narrow screen width.
Section 1.3 : Trig Functions
5. Determine the exact value of tan(3π4) without using a calculator.
Show All Steps Hide All Steps
Hint : Even though a unit circle only tells us information about sine and cosine it is still useful for tangents so sketch a unit circle and relate the angle to one of the standard angles in the first quadrant.
First we can notice that π−π4=3π4 and so (remembering that negative angles are rotated clockwise) we can see that the terminal line for 3π4 will form an angle of π4 with the negative x-axis in the second quadrant and we’ll have the following unit circle for this problem.
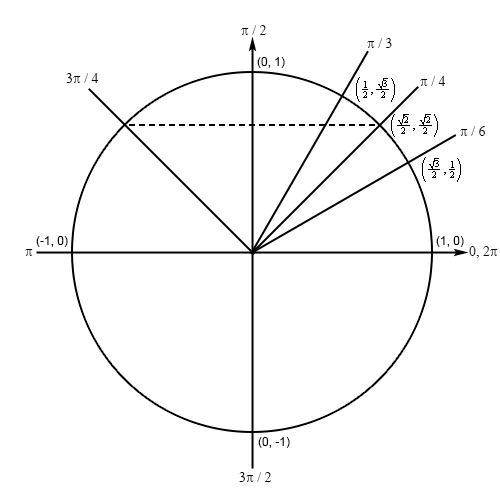
Hint : Given the obvious symmetry in the unit circle relate the coordinates of the line representing 3π4 to the coordinates of the line representing π4 and and then recall how tangent is defined in terms of sine and cosine to answer the question.
The coordinates of the line representing 3π4 will be the same as the coordinates of the line representing π4 except that the x coordinate will now be negative. So, our new coordinates will then be (−√22,√22) and so the answer is,
tan(3π4)=sin(3π4)cos(3π4)=√2/2−√2/2=−1