Section 2.9 : Continuity
2. The graph of \(f\left( x \right)\) is given below. Based on this graph determine where the function is discontinuous.
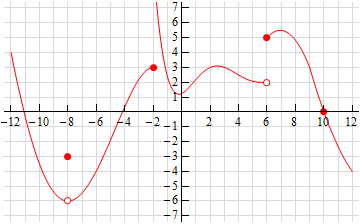
Before starting the solution recall that in order for a function to be continuous at \(x = a\) both \(f\left( a \right)\) and \(\mathop {\lim }\limits_{x \to a} f\left( x \right)\) must exist and we must have,
\[\mathop {\lim }\limits_{x \to a} f\left( x \right) = f\left( a \right)\]Using this idea it should be fairly clear where the function is not continuous.
First notice that at \(x = - 8\) we have,
\[\mathop {\lim }\limits_{x \to - {8^ - }} f\left( x \right) = - 6 = \mathop {\lim }\limits_{x \to - {8^ + }} f\left( x \right)\]and therefore, we also know that \(\mathop {\lim }\limits_{x \to - 8} f\left( x \right) = - 6\). We can also see that \(f\left( { - 8} \right) = - 3\) and so we have,
\[ - 6 = \mathop {\lim }\limits_{x \to - 8} f\left( x \right) \ne f\left( { - 8} \right) = - 3\]Because the function and limit have different values we can conclude that \(f\left( x \right)\) is discontinuous at \(x = - 8\).
Next let’s take a look at \(x = - 2\) we have,
\[\mathop {\lim }\limits_{x \to - {2^ - }} f\left( x \right) = 3 \ne \infty = \mathop {\lim }\limits_{x \to - {2^ + }} f\left( x \right)\]and therefore, we also know that \(\mathop {\lim }\limits_{x \to \, - 2} f\left( x \right)\) doesn’t exist. We can therefore conclude that \(f\left( x \right)\) is discontinuous at \(x = - 2\) because the limit does not exist.
Finally let’s take a look at \(x = 6\). Here we can see we have,
\[\mathop {\lim }\limits_{x \to {6^ - }} f\left( x \right) = 2 \ne 5 = \mathop {\lim }\limits_{x \to {6^ + }} f\left( x \right)\]and therefore, we also know that \[\mathop {\lim }\limits_{x \to \,6} f\left( x \right)\] doesn’t exist. So, once again, because the limit does not exist, we can conclude that \(f\left( x \right)\) is discontinuous at \(x = 6\).
All other points on this graph will have both the function and limit exist and we’ll have \(\mathop {\lim }\limits_{x \to a} f\left( x \right) = f\left( a \right)\) and so will be continuous.
In summary then the points of discontinuity for this graph are : \(x = - 8\), \(x = - 2\) and \(x = 6\).